Research interests
Differential geometry • Hyperbolic geometry • Moduli Spaces • Discrete Geometry • Math programming and visualization • Data Science and Machine Learning
Publications
Articles
-
The complex symplectic geometry of the deformation space of complex projective structures.
Geometry & Topology 19 (2015), no. 3, 1737–1775. Download. -
Minimal surfaces and symplectic structures of moduli spaces.
Geometriae Dedicata 175 (2015), 309–322. Download. -
Bi-Lagrangian structures and Teichmüller theory (with A. Sanders).
Preprint: arXiv:1708.09145 or HAL-01579284. Download. -
Computing discrete equivariant harmonic maps (with J. Gaster and L. Monsaingeon).
Preprint: arXiv:1810.11932 or HAL-02054982. Download. -
Computing harmonic maps between Riemannian manifolds (with J.Gaster and L. Monsaingeon).
Accepted in Canadian Journal of Mathematics (2022). Download. -
The sum of Lagrange numbers (with J. Gaster).
Proceedings of the AMS 149 (2021), 5385-5391. Download. -
Harmonic maps from Kähler manifolds.
Preprint: arXiv:2010.03545 or HAL-02961444. Download. -
Hyper-Kähler geometry of the Taubes moduli space (with F. Bonsante,
A. Sanders, and A. Seppi).
In preparation.
Notes
-
Higgs bundles and Hitchin components.
Notes for the workshop Higher Teichmüller-Thurston spaces in Paris XI, France in 2012. Download. -
Minimal surfaces and quasi-Fuchsian structures.
Notes for the NSF workshop Higgs bundles and harmonic maps in Asheville, NC in Januray 2015. Download. -
Hyperbolic geometry and applications.
Report of the GRG group (HITS). HITS Annual Report 2020.
Book
- Hyperbolic Geometry. → Book section.
PhD thesis
-
The symplectic geometry of complex projective structures.
Advisor: Prof. Jean-Marc Schlenker. View/Download.
Computational mathematics
Heidelberg Experimental Geometry Lab (HEGL)
Within the Research Station: Geometry & Dynamics, I was in charge of setting up the Lab (HEGL). The goal of HEGL is to promote the interaction between theoretical math and computational experimentation and visualization. HEGL provides advanced technology and hosts many student research projects.
→ HEGL website.
![]()
Circle Packings
This cross-platform computer software created by Benjamin Beeker and myself computes and shows circle packings and Riemann conformal mappings.
Harmony
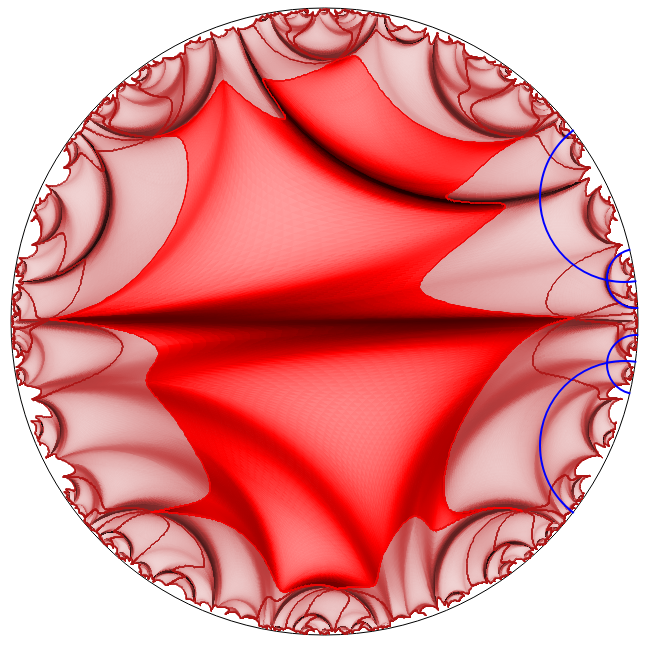
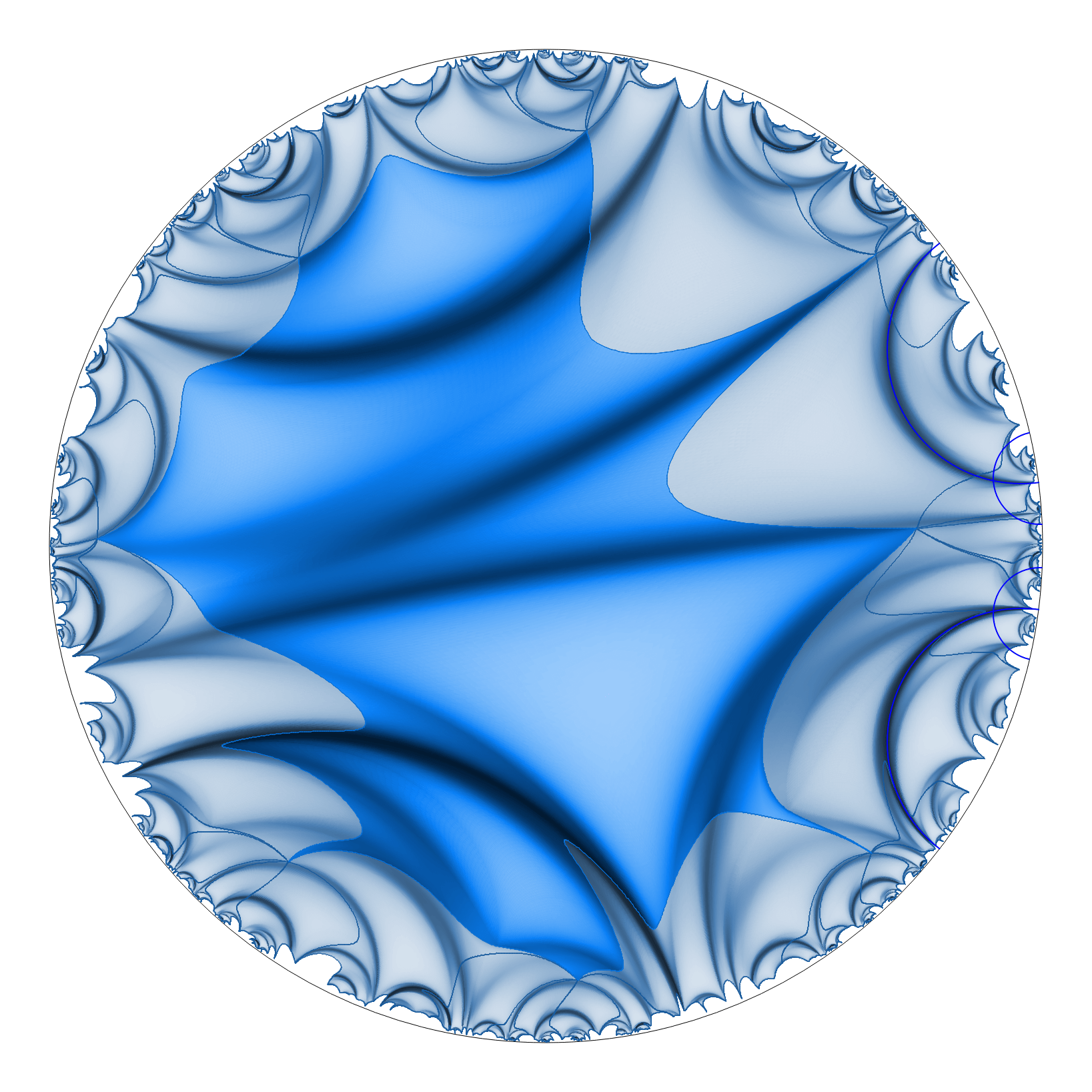
This cross-platform computer software created with Jonah Gaster computes and shows equivariant harmonic maps from the hyperbolic plane \(\mathbb{H}^2\) to hyperbolic 3-space \(\mathbb{H}^3\).
Mentoring student research
- I mentored the Master research project of T. Favier in the Summer 2021. Title: Hyperbolic geometry and graph embeddings.
- I mentored the Master thesis of Darja Zierau in the Summer 2019. Title: Cross-ratios of torsion points on elliptic curves.
- I mentor many undergraduate research projects at HEGL. → HEGL website.